Wide-band Continuum tests at 22-24 Ghz

Test observations were done January 8, 2004 to estimate continuum performance.
Project Name TGBT03C_002_02
Object 3C286
Scans 11-16 Focus and Peak
Scans 17-56 Total power nods, 30 sec each scan.

Result of peak scan on 3C286

  • Tsys = 34 K
  • Peak antenna temperature = 4.0 K
  • Gain = 1.7 K/Jy
    (using S(23GHz) = 2.375 JY for 3C286 (from Ott et al))

    Observations

    Scans 17-56 were a series of nodding scans, putting the calibrator 2C386 alternately in beam 3 and 4. Each scan was 30 seconds in length consisting of 1-second integrations.

    The DCR (digital continuum receiver) recorded two IF bands of 800 MHz bandwidth from each of the two beams and using two polarizations, hence 8 channels total. Due to an error in configuration, beam 3 was observing a band centered at 23 Ghz, and beam 4 at 24 GHz. The intention had been for each beam to observe two frequencies. Nevertheless, the setup is summarized in the following table.
    rx DCR_channel Pol/feed center_freq Tcal
    1 9 L3 23 GHz 5.15 K
    2 10 L4 24 GHz 3.18 K
    3 11 L3 23 GHz 5.15 K
    4 12 L4 24 GHz 3.18 K
    5 13 R3 23 GHz 5.27 K
    6 14 R4 24 GHz 5.01 K
    7 15 R3 23 GHz 5.27 K
    8 16 R4 24 GHz 5.01 K
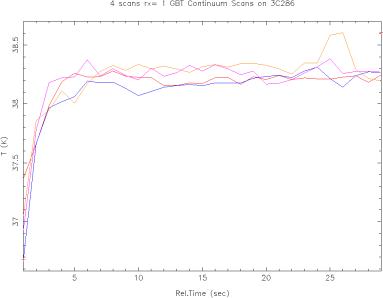
    Several typical 30-second scans on the calibrator 3C286 are plotted in the following graph.

    One may note that the antenna does not settle on the source until 5 seconds or so after the data taking starts. Therefore, we will eliminate the first 6 seconds of data from all scans for the analysis.

    Analysis

    There are several contribution to noise-like fluctuations that affect continuum observations. The total rms noise, dTtotal, includes components:
    dTtotal^2 = dTr^2 + dTa^2 + dTg^2
    in which
    Radiometer noise For and 800 MHz bandwidth and single polarization, the radiometer equation gives dTr = 1.3 mK for integration time Tint = 1 sec, and dTr = 0.4 mK for Tint=10 sec.

    Atmospheric noise To estimate the atmospheric term (dTa) we take the data for each 30-second scan, convert to units of temperature, and determine the rms residuals to a linear fit. The linear fit simulates the effect of baseline removal or beam switching at a few second rate. For off-source scans, this rms is an estimate of atmospheric fluctuations; for on-source scans, it includes the additional effects due to a strong source.
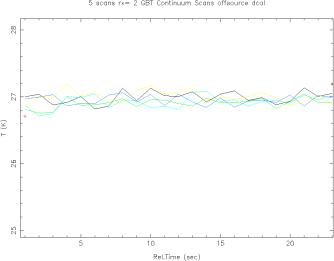
    Some typical blank sky scans are illustrated in the next graph, (Linear fit not removed).

    Gain fluctuations. To estimate the gain fluctuations, we look at the difference between the noise-cal-on and noise-cal-off phases. The calibration noise source is injected before the first amplifier stage and is switched on and off at a 1 Hz rate. The DCR integrates the power from these two phases separately. Thus for each integration we can look at the "delta_cal" or the difference between the cal-on and cal-off phases, and knowing the value of the calibration (Tcal) in temperature units, one can convert this difference to temperature units. The delta-cal is proportional to the gain (i.e., the conversion between counts and temperature). The rms of the delta_cal over a scan gives an estimate of the gain fluctuations.
    Specifically: dTg = Tsys * rms(delta_cal) / median(delta_cal)
    The following graph shows Tsys * delta_cal / median(delta_cal) for a few typical scans. The plot is for beam 3, offsource.

    It is of interest to note that beam 4 seems to perform considerably better than beam 3. The next graph shows the similar plot for beam #4.

    Conclusions: RMS fluctuations vs integration time.

    The statistics described above (dTa and dTg) were determined for individual scans, i.e., for integration time of one second. Also the quantities were computed for the average of 2, 5, 10, and 20 scans, to give results for integration times of 2, 5, 10, and 20 seconds.

    Since beam 3 appears to be excessively noisy, we will consider only beam 4 and will look at the off-source data. This will give results relevant to detecting faint sources. The results, with rms temperatures in milli-Kelvin (mK) are summarized as follows.
    statistics for beam 4
    Tintegration dTa (mK) dTg (mK)
    1 sec 13 83
    2 sec 8.6 65
    5 sec 6.0 43
    10 sec 3.9 27
    20 sec 2.0 20
    The rms fluctuations decrease approximately as the square root of the integration time. One can see that the gain fluctuations (dTg) dominate. Both dTg and dTa are considerably in excess of dTr, which is about 1.3 mK for Tint=1 sec. Because the beam switch follows the first amplifier, one cannot reduce the gain fluctuations by beam switching.