K-band Continuum tests: February 15, 2004

(F.Ghigo, April 28, 2004)
In these tests, we evaluate the noise fluctuations during 20-minute scans on blank sky, to estimate continuum performance. Both the "high band", 22-26 GHz, and the "low band", 18-22 GHz were studied.

Summary

The main results.

Introduction

The system was set up to record both beams in 2 spectral windows using the DCR inputs from the analog filter rack 800 MHz filters. Also the full band (~4GHz) was recorded with the DCR inputs from the IF Rack. Thus, the DCR recorded four wide-band channels detected in the IF Rack (two polarizations, two beams), and eight 800 MHz bandwidth channels from the Analog Filter Rack (two frequency bands, two polarizations, two beams). The 800 MHz channels have passed through many more devices including two more mixing stages than the signals detected in the IF Rack. Thus we can check whether the latter stages of the IF system contributes much noise.

Table 1: IF Channels. The "Name" gives the labels that will identify these channels on the subsequent plots. The name is the beam designation (L1, R1, L2, R2, etc) and "_wide" for the wideband IFs from the IF Rack, or the center frequency for the 800 MHz channels. For example, "L4_25" means beam 4, left circular, 800 MHz band centered at 25 GHz.
18-22 GHz Receiever   22-26 GHz Receiever
Name DCR
channel 		Fcent BW(MHz)   Name DCR
channel 		Fcent BW(MHz)
L1_wide 1 19.9 4200   L3_wide 2 24.2 4800
R1_wide 3 19.9 4200   R3_wide 4 24.2 4800
L2_wide 5 19.9 4200   L4_wide 6 24.2 4800
R2_wide 7 19.9 4200   R4_wide 8 24.2 4800
L1_19 9 19.0 800   L4_24 9 24.0 800
L1_20 10 20.0 800   L4_25 10 25.0 800
L2_19 11 19.0 800   L3_24 11 24.0 800
L2_20 12 20.0 800   L3_25 12 25.0 800
R1_19 13 19.0 800   R4_24 13 24.0 800
R1_20 14 20.0 800   R4_25 14 25.0 800
R2_19 15 19.0 800   R3_24 15 24.0 800
R2_20 16 20.0 800   R3_25 16 25.0 800

Tsys plots.

In this set of plots, we show the data scaled to units of Temperature. The data sampling period was 0.1 second, with noise cal switching. We have taken the average cal deflection for the whole scan and the average Tcal value to scale the data to units of Kelvin.

Figure 1. Wide-band channels, 22-26 GHz

800 MHz channels, 22-26 GHz
Spectra have been shifted arbitrary amounts in Y, for clarity.
These are pretty much the same as the wide-band channels, but the Y scale is different.

Figure 2. 800MHz channels, 22-26 GHz

Figure 3. Wide-band channels, 18-22 GHz

Figure 4. 800 MHz channels, 18-22 GHz

Again, spectra have been arbitrarily shifted in Y.

Gain Fluctuations

To consider gain fluctuations, we have looked at the power difference beteween the noise cal on and noise cal off, and have plotted this difference in the following graphs. These differences have been scaled to units of Kelvins.

One may note a systematic drift in some channels over the 30 minute observaion.

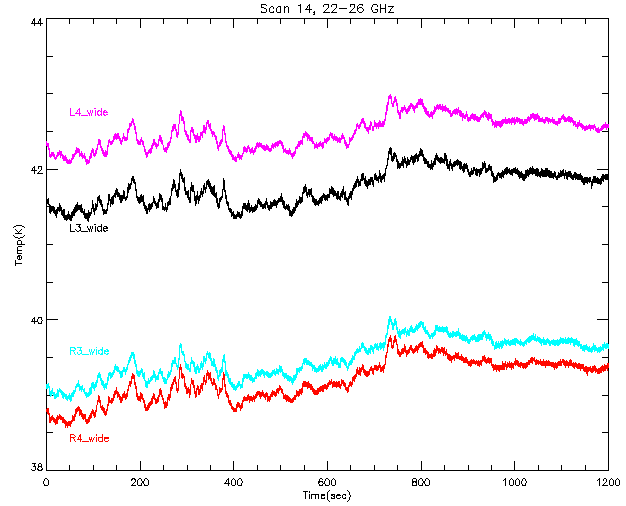
Figure 5. Gain Fluctuations: 22-26 GHz (wideband)

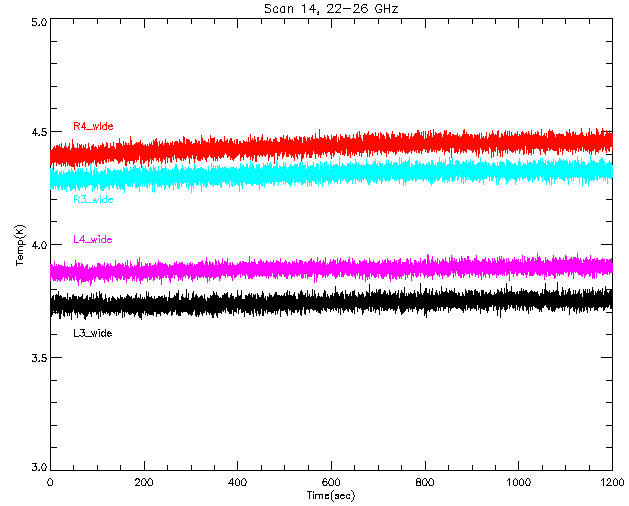
The drift in the gain is characterized by taking the difference between an average of points at the beginning of the scan and at the end, then dividing by the average over the whole scan. This is shown in the "Drift" column in Table 2. The gain rms values ("Grms") are the rms over the scan after removing a 2nd order polynomial fit to the data. We would expect the rms noise to be about a factor of two lower for the 4 GHz bandwidth channel, but it isn't. As one can see from the table, the wideband channels have rms of about 16-20 milliKelvins for the 0.1 sec sample time, and the 800 MHz channels are about 30 mK.

The results for longer integration times were found by averaging the raw data over 1, 10, and 100 seconds and doing the same analysis that was done for the 0.1 sec data. The results are shown in Table 2. Similar operations were done for the low band receiver (18-22 GHz) and those results are shown below in Table 3.

One may compare these results with those from the January 8 data, we find the rms gain fluctuations much smaller, but still considerably larger than the prediction of the radiometer equation. The radiometer equation predictions are listed at the end of the table.

Table 2. Results for 22-26 GHz (scan #14). 
 Channel  TCal    Tsys    Drift   ----------Grms(mK)---------------
           K       K       %      (0.1 s)  (1.0 s)  (10 s)  (100 s)
 L3_wide  3.74   41.76    0.630    18.85    5.72     2.71    1.91
 R3_wide  4.31   39.49    0.994    19.61    5.66     2.22    1.16
 L4_wide  3.89   42.49    0.706    16.49    4.93     2.16    1.48
 R4_wide  4.43   39.19    1.409    19.48    5.68     2.36    1.45
 L4_24    3.45   40.81    0.724    27.24    8.83     3.37    1.71
 L4_25    3.44   39.04    0.699    24.18    8.40     5.21    4.45
 L3_24    3.48   41.04    0.460    27.95    8.63     3.71    2.06
 L3_25    3.09   34.85    0.292    23.05    7.33     2.83    1.32
 R4_24    4.72   38.99    1.135    30.61    9.12     3.55    1.54
 R4_25    4.38   37.04   -0.371    26.34    8.11     3.17    1.18
 R3_24    4.39   44.08    0.701    29.81    9.01     3.76    2.37
 R3_25    4.02   34.89    0.482    23.16    7.27     3.05    1.68


 Predicted (bw=4800 MHz; Tsys=40)  1.7      0.5      0.2     0.05
 Predicted (bw=800 MHz;  Tsys=40)  4.1      1.3      0.4     0.13

Figure 6. Gain Fluctuations: 18-22 GHz (wideband)

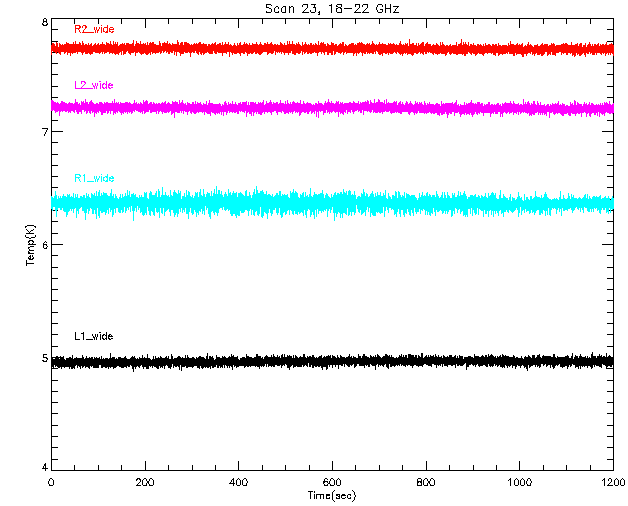
Table 3. Results for 18-22 GHz (scan #23). 
 Channel  TCal    Tsys    Drift   ----------Grms(mK)---------------
           K       K       %      (0.1 s)  (1.0 s)  (10 s)  (100 s)
 L1_wide  5.16   35.93    0.261    20.46    6.11     2.60    1.30
 R1_wide  5.37   37.94   -0.182    35.94   10.54     3.78    1.69
 L2_wide  5.21   35.74   -0.275    21.28    6.95     3.89    2.97
 R2_wide  4.73   39.05   -0.123    20.20    5.98     2.54    1.67
 L1_19    4.61   32.01   -0.362    19.67    6.24     2.71    1.77
 L1_20    5.89   32.30    0.063    20.67    7.41     4.36    3.19
 L2_19    4.64   32.22   -0.673    20.63    6.48     3.04    2.13
 L2_20    5.92   32.25   -0.647    21.76    7.57     4.57    3.32
 R1_19    6.62   38.65   -0.762    35.87   10.79     4.22    1.79
 R1_20    5.36   38.47   -1.015    37.32   11.12     4.40    2.11
 R2_19    5.86   26.13    0.249    20.95    7.10     3.85    2.61
 R2_20    3.17   24.37    0.536    19.54    6.01     2.57    1.35

 Predicted (bw=4200 MHz; Tsys=40)  1.8      0.4      0.2     0.06
 Predicted (bw=800 MHz;  Tsys=40)  4.1      1.3      0.4     0.13
We may note here that the R1 channel is significantly noisier than the others.

Removing Atmospheric fluctuations.

We try to remove atmospheric fluctuations by dividing the data from one beam by the other. First, for the 18-22 GHz data, we divide beam 1 by from beam 2. The result has been scaled to a mean Tsys of 40 K. A plot of these scans shows drifts over time scales of minutes.

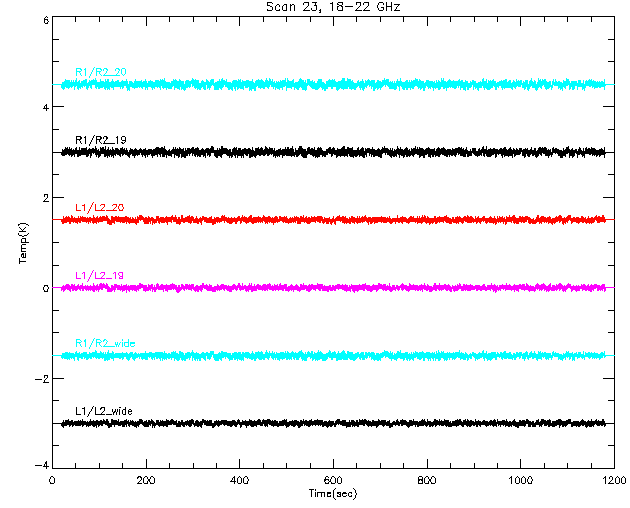
Figure 7. Beam Ratios: 18-22 GHz

To remove these long-term drifts, we median-filtered the data and subtracted from the original, then deleted outliers beyond 3 sigma. (The median filter window was 1/30 of the scan duration). The result is a scan with flat baselines. The next plot shows these flattened scans. Arbitrary offsets have been added in Y so the plots are not all on top of each other.

Figure 8. Flattened Beam Ratios: 18-22 GHz

This table shows the RMS in each channel after dividing and flattening. The RMS is show for three cases integrating over 0.1, 1.0, and 10 seconds and doing the same processing after doing the integrating in each case.

Table 4. RMS for beam ratios, 18-22 GHz. 

Channel      --------Trms(mK)-------------
             (0.1 s)  (1.0 s)  (10 s)
 L1/L2(wide)   30.46   19.67     9.20   
 R1/R2(wide)   36.74   22.67    10.07  
 L1/L2(19)     31.41   20.54    11.27  
 L1/L2(20)     32.45   21.09    10.05
 R1/R2(19)     42.27   26.14    11.85
 R1/R2(20)     44.57   28.23    13.74
We would expect that the ratio of data from the two beams would show fluctuations due mostly to gain variations; atmospheric effects should have been removed. The combination of the two beams would result in an rms that is about sqrt(2) larger than the rms of the individual beam. This seems to be approximately right for the 0.1 sec sampled data. But for the longer integrations, the RMS is not decreasing as fast as expected.

For 22-26 GHz band, divide beam 3 data by beam 4, then calculate the rms over after removing drift by a median filter, and removing outliers.

Table 5. RMS beam ratios, 22-26 GHz. 

Channel      --------Trms(mK)-------------
             (0.1 s)  (1.0 s)  (10 s)
 L3/L4(wide)  27.22    18.93    9.25
 R3/R4(wide)  26.60    16.90    9.67
 L3/L4(24)    32.53    21.14   10.61
 L3/L4(25)    26.70    17.55    9.20
 R3/R4(24)    36.32    21.06   10.56
 R3/R4(25)    27.64    16.16    8.94

RMS vs Integration time.

The results are summarized in Figure 9. The red circles show the rms for the beam ratio L1/L2 wide band channel (Table 4), and the black stars show the gain rms for L1_wide channel (Table 3). These plots are representative of all the data. There is not much difference between the different IF channels (except for R1) and not much difference between the 18-22 and 22-26 GHz bands. The gain rms falls off not as the square root of the integration time (T), but a little slower, as T**-0.4. The beam ratio falls off even slower, as T**-0.26.

Figure 9. RMS vs Integration time: 18-22 GHz